Difference between revisions of "Diffuse scattering"
(→Analysis) |
KevinYager (talk | contribs) (→See Also) |
||
| (39 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
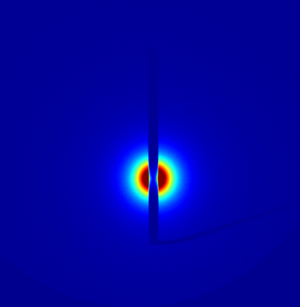
| − | '''Diffuse scattering''' is the scattering that arises from any departure of the material structure from that of a perfectly regular [[lattice]]. One can think of it as the signal that arises from disordered structures, and it appears in experimental data as scattering spread over a wide ''q''-range (diffuse). Diffuse scattering is generally difficult to quantify, because of the wide variety of effects that contribute to it. | + | [[Image:Diffuse scattering example SAXS.png|300px|thumb|right|Example [[SAXS]] of a disordered composite, which generates [[diffuse scattering]] at low ''[[q]]''.]] |
| + | |||
| + | '''Diffuse scattering''' is the [[scattering]] that arises from any departure of the material structure from that of a perfectly regular [[lattice]]. One can think of it as the signal that arises from disordered structures, and it appears in experimental data as scattering spread over a wide ''q''-range (diffuse). Diffuse scattering is generally difficult to quantify, because of the wide variety of effects that contribute to it. | ||
Bragg diffraction occurs when scattering amplitudes add ''constructively''. If there is a defect in a crystal lattice (e.g. atom missing or in a slightly 'wrong' position), then the amplitude of the Bragg peak decreases. This 'lost' scattering intensity is redistributed into diffuse scattering. The diffuse scattering thus arises from the local (short range) configuration of the material (not the long-range structural order). | Bragg diffraction occurs when scattering amplitudes add ''constructively''. If there is a defect in a crystal lattice (e.g. atom missing or in a slightly 'wrong' position), then the amplitude of the Bragg peak decreases. This 'lost' scattering intensity is redistributed into diffuse scattering. The diffuse scattering thus arises from the local (short range) configuration of the material (not the long-range structural order). | ||
| Line 6: | Line 8: | ||
==Causes== | ==Causes== | ||
| + | This is only a partial list of sources of diffuse scattering: | ||
* '''Thermal motion''' causes atoms to jitter about their ideal unit cell positions, which decorelates them. This suppresses the intensity of the Bragg peaks, especially the higher-order peaks (see [[Debye-Waller factor]]), and instead generates high-''q'' diffuse scattering. (One can also think of this in terms of phonons: in ordered systems the diffuse scattering is probing phonon modes.) | * '''Thermal motion''' causes atoms to jitter about their ideal unit cell positions, which decorelates them. This suppresses the intensity of the Bragg peaks, especially the higher-order peaks (see [[Debye-Waller factor]]), and instead generates high-''q'' diffuse scattering. (One can also think of this in terms of phonons: in ordered systems the diffuse scattering is probing phonon modes.) | ||
* '''Static disorder''' in crystals (vacancy defects, substitutional defects, stacking faults, etc.) similarly creates diffuse scattering. | * '''Static disorder''' in crystals (vacancy defects, substitutional defects, stacking faults, etc.) similarly creates diffuse scattering. | ||
| Line 11: | Line 14: | ||
* '''Nanoscale disorder''' gives rise to low-''q'' diffuse scattering. For instance, a disordered polymer blend (or a bulk heterojunction) or a random packing of nanoparticles, will generate substantial low-''q'' diffuse scattering. | * '''Nanoscale disorder''' gives rise to low-''q'' diffuse scattering. For instance, a disordered polymer blend (or a bulk heterojunction) or a random packing of nanoparticles, will generate substantial low-''q'' diffuse scattering. | ||
* '''Surface roughness''' in thin films measured by [[GISAXS]] gives rise to low-''q'' diffuse scattering in GISAXS. Roughness will tend to broaden (and increase the intensity of) the specular rod, and will also generate intense low-''q'' scattering. | * '''Surface roughness''' in thin films measured by [[GISAXS]] gives rise to low-''q'' diffuse scattering in GISAXS. Roughness will tend to broaden (and increase the intensity of) the specular rod, and will also generate intense low-''q'' scattering. | ||
| − | * '''Polymer chains in solution''' generate scattering without a well-defined size-scale. This is normally interpreted in terms of the [[form factor]] of the polymer chain. However one can also think of it as the polymer chains having disordered arrangements and thus giving rise to diffuse scattering | + | * '''Particle size/shape polydispersity''' introduces a diffuse [[background]]. |
| + | * '''Polymer chains in solution''' generate scattering without a well-defined size-scale. This is normally interpreted in terms of the [[form factor]] of the polymer chain. However one can also think of it as the polymer chains having disordered arrangements and thus giving rise to diffuse scattering (c.f. [[definitional boundaries]]). [[Example:Polymer clustering|Polymer clustering or gelation]] can also give rise to diffuse-like scattering. | ||
==Analysis: low-''q''== | ==Analysis: low-''q''== | ||
| Line 17: | Line 21: | ||
===Ornstein-Zernike model=== | ===Ornstein-Zernike model=== | ||
| − | Yields correlation length (ξ): | + | Yields correlation length (''ξ''): |
::<math> | ::<math> | ||
I(q) \propto \frac{1}{ 1 + q^2 \xi^2 } | I(q) \propto \frac{1}{ 1 + q^2 \xi^2 } | ||
</math> | </math> | ||
* References: | * References: | ||
| − | ** M. Kahlweit , R. Strey , P. Firman "[http://pubs.acs.org/doi/abs/10.1021/j100276a038 Search for tricritical points in ternary systems: water-oil-nonionic amphiphile]" ''J. Phys. Chem. 1986, 90, 4, 674. [http://dx.doi.org/10.1021/j100276a038 doi: 10.1021/j100276a038] | + | ** M. Kahlweit , R. Strey , P. Firman "[http://pubs.acs.org/doi/abs/10.1021/j100276a038 Search for tricritical points in ternary systems: water-oil-nonionic amphiphile]" ''J. Phys. Chem.'' 1986, 90, 4, 674. [http://dx.doi.org/10.1021/j100276a038 doi: 10.1021/j100276a038] |
| − | ** M. Teubner and R. Strey "[http://scitation.aip.org/content/aip/journal/jcp/87/5/10.1063/1.453006 Origin of the scattering peak in microemulsions]" '' J. Chem. Phys. 1987, 87, 3195. [http://dx.doi.org/10.1063/1.453006 doi: 10.1063/1.453006] | + | ** M. Teubner and R. Strey "[http://scitation.aip.org/content/aip/journal/jcp/87/5/10.1063/1.453006 Origin of the scattering peak in microemulsions]" '' J. Chem. Phys.'' 1987, 87, 3195. [http://dx.doi.org/10.1063/1.453006 doi: 10.1063/1.453006] |
** U. Nellen, J. Dietrich, L. Helden, S. Chodankar, K. Nygård, J. Friso van der Veenbc, C. Bechingerad "[http://pubs.rsc.org/en/Content/ArticleLanding/2011/SM/c1sm05103b#!divAbstract Salt-induced changes of colloidal interactions in critical mixtures]" ''Soft Matter'' 2011, 7, 5360. [http://dx.doi.org/10.1039/c1sm05103b doi: 10.1039/c1sm05103b] | ** U. Nellen, J. Dietrich, L. Helden, S. Chodankar, K. Nygård, J. Friso van der Veenbc, C. Bechingerad "[http://pubs.rsc.org/en/Content/ArticleLanding/2011/SM/c1sm05103b#!divAbstract Salt-induced changes of colloidal interactions in critical mixtures]" ''Soft Matter'' 2011, 7, 5360. [http://dx.doi.org/10.1039/c1sm05103b doi: 10.1039/c1sm05103b] | ||
| Line 34: | Line 38: | ||
* References: | * References: | ||
** P. Debye, A.M. Bueche "[http://scitation.aip.org/content/aip/journal/jap/20/6/10.1063/1.1698419 Scattering by an Inhomogeneous Solid]" ''J. Appl. Phys.'' 1949, 20, 518. [http://link.aip.org/link/doi/10.1063/1.1698419 doi: 10.1063/1.1698419] | ** P. Debye, A.M. Bueche "[http://scitation.aip.org/content/aip/journal/jap/20/6/10.1063/1.1698419 Scattering by an Inhomogeneous Solid]" ''J. Appl. Phys.'' 1949, 20, 518. [http://link.aip.org/link/doi/10.1063/1.1698419 doi: 10.1063/1.1698419] | ||
| − | ** P. Debye, H.R. Anderson, H. Brumberger "[ Scattering by an Inhomogeneous Solid. II. The Correlation Function and Its Application]" ''J. Appl. Phys.'' 1957, 28, 679. [http://link.aip.org/link/doi/10.1063/1.1722830 doi: 10.1063/1.1722830] | + | ** P. Debye, H.R. Anderson, H. Brumberger "[http://scitation.aip.org/content/aip/journal/jap/28/6/10.1063/1.1722830 Scattering by an Inhomogeneous Solid. II. The Correlation Function and Its Application]" ''J. Appl. Phys.'' 1957, 28, 679. [http://link.aip.org/link/doi/10.1063/1.1722830 doi: 10.1063/1.1722830] |
** [http://www.ncnr.nist.gov/resources/sansmodels/DAB.html NCNR page] | ** [http://www.ncnr.nist.gov/resources/sansmodels/DAB.html NCNR page] | ||
| − | ===Guinier model=== | + | ===[[Guinier plot|Guinier]] model=== |
| − | Yields average | + | Yields average radius of gyration (''R<sub>g</sub>''): |
| + | ::<math> | ||
| + | I(q) \propto e^{ - R_g^2 q^2 / 3 } | ||
| + | </math> | ||
| + | |||
| + | * References: | ||
| + | ** B. Hammouda [http://journals.iucr.org/j/issues/2010/04/00/ce5078/ A new Guinier-Porod model] ''J. Appl. Cryst. '''2010''', 43, 716-719. [http://dx.doi.org/10.1107/S0021889810015773 doi: 10.1107/S0021889810015773] | ||
| + | |||
| + | ===Additional=== | ||
| + | * G. Beaucage [http://scripts.iucr.org/cgi-bin/paper?S0021889895005292 Approximations Leading to a Unified Exponential/Power-Law Approach to Small-Angle Scattering] ''J. Appl. Cryst.'' '''1995''', 28, 717-728. [http://dx.doi.org/10.1107/S0021889895005292 doi: 10.1107/S0021889895005292][ | ||
| + | * G. Beaucage [http://scripts.iucr.org/cgi-bin/paper?S0021889895011605 Small-Angle Scattering from Polymeric Mass Fractals of Arbitrary Mass-Fractal Dimension] ''J. Appl. Cryst.'' '''1996''', 29, 134-146. [http://dx.doi.org/10.1107/S0021889895011605 doi: 10.1107/S0021889895011605] | ||
| + | * B. Hammouda [http://journals.iucr.org/j/issues/2010/04/00/ce5078/ A new Guinier-Porod model] ''J. Appl. Cryst. '''2010''', 43, 716-719. [http://dx.doi.org/10.1107/S0021889810015773 doi: 10.1107/S0021889810015773] | ||
==Analysis: high-''q''== | ==Analysis: high-''q''== | ||
| − | ===Porod law=== | + | ===[[Porod scattering|Porod]] law=== |
| − | + | For high-''q'', gives specific surface area (''S''): | |
::<math> | ::<math> | ||
I(q) \propto S q^{-4} | I(q) \propto S q^{-4} | ||
</math> | </math> | ||
| − | * | + | * References: |
| − | ** | + | ** W. Ruland "[http://scripts.iucr.org/cgi-bin/paper?S0021889871006265 Small-angle scattering of two-phase systems: determination and significance of systematic deviations from Porod's law]" ''J. Appl. Cryst.'' 1971, 4, 70. [http://dx.doi.org/10.1107/S0021889871006265 doi: 10.1107/S0021889871006265] |
| − | + | ** J. T. Koberstein, B. Morra and R. S. Stein "[http://scripts.iucr.org/cgi-bin/paper?S0021889880011478 The determination of diffuse-boundary thicknesses of polymers by small-angle X-ray scattering]" ''J. Appl. Cryst.'' 1980, 13, 34. [http://dx.doi.org/10.1107/S0021889880011478 doi: 10.1107/S0021889880011478] | |
| − | ** J. T. Koberstein, B. Morra and R. S. Stein [http:// | + | ** [http://en.wikipedia.org/wiki/Porod%27s_law Wikipedia: Porod law] |
| + | |||
===Porod fractal law=== | ===Porod fractal law=== | ||
| − | + | For high-''q'', gives specific surface area: | |
:: <math>\lim_{q \rightarrow \infty} I(q) \propto S' q^{-(6-d)}</math> | :: <math>\lim_{q \rightarrow \infty} I(q) \propto S' q^{-(6-d)}</math> | ||
| − | * | + | * References: |
| − | ** [http://en.wikipedia.org/wiki/Porod%27s_law Wikipedia] | + | ** [http://en.wikipedia.org/wiki/Porod%27s_law Wikipedia: Porod law] |
| + | |||
| + | ==Structured Diffuse Scattering== | ||
| + | Even the highest-quality single-crystal materials exhibit some level of disorder, giving rise to diffuse scattering. Single-Crystal Diffuse Scattering (SCDS) can be analyzed to understand local (short-range) ordering. Experimentally, it appears as weak and diffuse 'lines' that interconnect between the bright diffraction spots. While the diffraction spots describe the idealized [[unit-cell]], this diffuse scattering encodes the deviation from the average structure. The diffuse scattering can be quantitatively modeled. | ||
| + | |||
| + | * T. R. Welberry and D. J. Goossens [http://journals.iucr.org/a/issues/2008/01/00/sc5007/ The interpretation and analysis of diffuse scattering using Monte Carlo simulation methods] ''Acta Crystallographica Section A: Foundations and Advances'' '''2008''', 64 (1), 23-32 [http://dx.doi.org/10.1107/S0108767307041918 doi: 10.1107/S0108767307041918] | ||
| + | * E. J. Chan, T. R. Welberry, D. J. Goossens, A. P. Heerdegen, A. G. Beasley and P. J. Chupas [http://journals.iucr.org/b/issues/2009/03/00/issconts.html Single-crystal diffuse scattering studies on polymorphs of molecular crystals. I. The room-temperature polymorphs of the drug benzocaine] ''Acta Crystallographica Section B Structural Science'' '''2009'', B65 (3), 382-392 [http://dx.doi.org/10.1107/S0108768109015857 doi: 10.1107/S0108768109015857] | ||
| + | * T. R. Welberry and D. J. Goossens [http://journals.iucr.org/m/issues/2014/06/00/zx5002/index.html Diffuse scattering and partial disorder in complex structures] ''IUCrJ'' '''2014''' 1 (6), 550-562 [http://dx.doi.org/10.1107/S205225251402065X doi: 10.1107/S205225251402065X] | ||
| + | * E. J. Chan [http://scripts.iucr.org/cgi-bin/paper?po5039 On the use of molecular dynamics simulation to calculate X-ray thermal diffuse scattering from molecular crystals] ''J. Appl. Cryst.'' '''2015''', 48. [http://dx.doi.org/10.1107/S1600576715013242 doi: 10.1107/S1600576715013242] | ||
| + | * A.G.A. Nisbet, G. Beutier, F. Fabrizi, B. Mosera and S. P. Collins [http://journals.iucr.org/a/issues/2015/01/00/td5022/index.html Diffuse multiple scattering] ''Acta Cryst. A'' '''2015''', 20-25. [http://dx.doi.org/10.1107/S2053273314026515 doi: 10.1107/S2053273314026515] | ||
| + | |||
| + | More broadly, highly anisotropic and structured diffuse scattering indicates that the material exhibits some non-trivial combination of order and disorder in realspace. For instance, the disorder/defects may be ordered in some way (correlated disorder). Whereas diffuse scattering is traditionally seen as encoding structuring on a very local scale, structured diffuse scattering relates to order on intermediate size-scales. | ||
| + | * Ray Withers [http://journals.iucr.org/m/issues/2015/01/00/gq5002/index.html A modulation wave approach to the order hidden in disorder] ''IUCrJ'' '''2015''', 2 (1), 74-84 [http://dx.doi.org/10.1107/S2052252514022556 doi: 10.1107/S2052252514022556] | ||
| + | * David A. Keen & Andrew L. Goodwin [http://www.nature.com/nature/journal/v521/n7552/full/nature14453.html The crystallography of correlated disorder] ''Nature'' '''2015''', 521, 303-309. [http://dx.doi.org/10.1038/nature14453 doi:10.1038/nature14453] | ||
| + | |||
| + | ==Liquid Surface Diffuse Scattering== | ||
| + | Grazing-incidence scattering can be performed on liquid interfaces. GISAXS scattering peaks may be seen if there is some kind of ordering at the interface (e.g. nanoparticles); but a pure liquid interface will, generically, not exhibit [[structure factor|structural scattering]]. However, the disorder of the interface will generate diffuse scattering. In particular, the interface will fluctuate due to thermally-induced waves: i.e. [[capillary waves]]. | ||
| + | * Oleg Shpyrko [http://www.aps.anl.gov/Users/Scientific_Interest_Groups/LSM/schedule/Shpyrko_Oct05.pdf Overview of X-ray Reflectivity and Diffuse Scattering from Liquid Surfaces] | ||
| + | * C. Fradin, A. Braslau, D. Luzet, D. Smilgies, M. Alba, N. Boudet, K. Mecke & J. Daillant [http://www.nature.com/nature/journal/v403/n6772/abs/403871a0.html Reduction in the surface energy of liquid interfaces at short length scales] ''Nature'' '''2000''', 403, 871-874. [http://dx.doi.org/10.1038/35002533 doi: 10.1038/35002533] | ||
| + | |||
| + | ==Nanoscale Diffuse Scattering== | ||
| + | * A. Fernández Herrero, M. Pflüger, J. Probst, F. Scholze and V. Soltwisch [http://scripts.iucr.org/cgi-bin/paper?rg5132 Characteristic diffuse scattering from distinct line roughnesses] ''J. Appl. Cryst.'' '''2017''', 50, 1766-1772. [https://doi.org/10.1107/S1600576717014455 doi: 10.1107/S1600576717014455] | ||
==See Also== | ==See Also== | ||
* [http://neutrons.ornl.gov/conf/nxs2011/pdf/lectures/Diffuse11-GeneIce.pdf Diffuse Scattering] from ORNL | * [http://neutrons.ornl.gov/conf/nxs2011/pdf/lectures/Diffuse11-GeneIce.pdf Diffuse Scattering] from ORNL | ||
* [http://www.neutron.ethz.ch/education/Lectures/neutronfall/Lecture_4-2 Diffuse scattering in crystals] from ETH Zurich | * [http://www.neutron.ethz.ch/education/Lectures/neutronfall/Lecture_4-2 Diffuse scattering in crystals] from ETH Zurich | ||
| + | * P. Ehrhart [http://www.sciencedirect.com/science/article/pii/0022311578902441 The configuration of atomic defects as determined from scattering studies] ''Journal of Nuclear Materials'' '''1978''', 6970, 200-214. [http://dx.doi.org/10.1016/0022-3115(78)90244-1 doi: 10.1016/0022-3115(78)90244-1] | ||
| + | * T. R. Welberry and D. J. Goossens [http://journals.iucr.org/m/issues/2014/06/00/zx5002/index.html Diffuse scattering and partial disorder in complex structures] ''IUCrJ'' '''2014''' 1 (6), 550-562 [http://dx.doi.org/10.1107/S205225251402065X doi: 10.1107/S205225251402065X] | ||
Latest revision as of 10:36, 1 December 2017
Diffuse scattering is the scattering that arises from any departure of the material structure from that of a perfectly regular lattice. One can think of it as the signal that arises from disordered structures, and it appears in experimental data as scattering spread over a wide q-range (diffuse). Diffuse scattering is generally difficult to quantify, because of the wide variety of effects that contribute to it.
Bragg diffraction occurs when scattering amplitudes add constructively. If there is a defect in a crystal lattice (e.g. atom missing or in a slightly 'wrong' position), then the amplitude of the Bragg peak decreases. This 'lost' scattering intensity is redistributed into diffuse scattering. The diffuse scattering thus arises from the local (short range) configuration of the material (not the long-range structural order).
In the limit of disorder, one entirely lacks a realspace lattice and thus scattering does not generate any Bragg peaks. However, a disordered structure will still give rise to diffuse scattering. The Fourier transform of a disordered structure will not give any well-defined peaks, but will give a distribution of scattering intensity over a wide range of q-values. Thus samples with an inherently disordered structure (polymer blends, randomly packed nanoparticles, etc.) will only generate diffuse scattering.
Contents
Causes
This is only a partial list of sources of diffuse scattering:
- Thermal motion causes atoms to jitter about their ideal unit cell positions, which decorelates them. This suppresses the intensity of the Bragg peaks, especially the higher-order peaks (see Debye-Waller factor), and instead generates high-q diffuse scattering. (One can also think of this in terms of phonons: in ordered systems the diffuse scattering is probing phonon modes.)
- Static disorder in crystals (vacancy defects, substitutional defects, stacking faults, etc.) similarly creates diffuse scattering.
- Grain structure in otherwise ordered materials will also contribute. The grains themselves can count as 'scattering objects', but since their size is ill-defined, the grain boundaries give rise to diffuse scattering.
- Nanoscale disorder gives rise to low-q diffuse scattering. For instance, a disordered polymer blend (or a bulk heterojunction) or a random packing of nanoparticles, will generate substantial low-q diffuse scattering.
- Surface roughness in thin films measured by GISAXS gives rise to low-q diffuse scattering in GISAXS. Roughness will tend to broaden (and increase the intensity of) the specular rod, and will also generate intense low-q scattering.
- Particle size/shape polydispersity introduces a diffuse background.
- Polymer chains in solution generate scattering without a well-defined size-scale. This is normally interpreted in terms of the form factor of the polymer chain. However one can also think of it as the polymer chains having disordered arrangements and thus giving rise to diffuse scattering (c.f. definitional boundaries). Polymer clustering or gelation can also give rise to diffuse-like scattering.
Analysis: low-q
Diffuse scattering can be difficult to quantify, since so many different effects contribute to it. Nevertheless, if one has a good understanding of the expected kind of disorder, one can fit the diffuse scattering with a model.
Ornstein-Zernike model
Yields correlation length (ξ):
- References:
- M. Kahlweit , R. Strey , P. Firman "Search for tricritical points in ternary systems: water-oil-nonionic amphiphile" J. Phys. Chem. 1986, 90, 4, 674. doi: 10.1021/j100276a038
- M. Teubner and R. Strey "Origin of the scattering peak in microemulsions" J. Chem. Phys. 1987, 87, 3195. doi: 10.1063/1.453006
- U. Nellen, J. Dietrich, L. Helden, S. Chodankar, K. Nygård, J. Friso van der Veenbc, C. Bechingerad "Salt-induced changes of colloidal interactions in critical mixtures" Soft Matter 2011, 7, 5360. doi: 10.1039/c1sm05103b
Debye-Bueche random two-phase model
Yields correlation length (a):
- References:
- P. Debye, A.M. Bueche "Scattering by an Inhomogeneous Solid" J. Appl. Phys. 1949, 20, 518. doi: 10.1063/1.1698419
- P. Debye, H.R. Anderson, H. Brumberger "Scattering by an Inhomogeneous Solid. II. The Correlation Function and Its Application" J. Appl. Phys. 1957, 28, 679. doi: 10.1063/1.1722830
- NCNR page
Guinier model
Yields average radius of gyration (Rg):
- References:
- B. Hammouda A new Guinier-Porod model J. Appl. Cryst. 2010, 43, 716-719. doi: 10.1107/S0021889810015773
Additional
- G. Beaucage Approximations Leading to a Unified Exponential/Power-Law Approach to Small-Angle Scattering J. Appl. Cryst. 1995, 28, 717-728. doi: 10.1107/S0021889895005292[
- G. Beaucage Small-Angle Scattering from Polymeric Mass Fractals of Arbitrary Mass-Fractal Dimension J. Appl. Cryst. 1996, 29, 134-146. doi: 10.1107/S0021889895011605
- B. Hammouda A new Guinier-Porod model J. Appl. Cryst. 2010, 43, 716-719. doi: 10.1107/S0021889810015773
Analysis: high-q
Porod law
For high-q, gives specific surface area (S):
- References:
- W. Ruland "Small-angle scattering of two-phase systems: determination and significance of systematic deviations from Porod's law" J. Appl. Cryst. 1971, 4, 70. doi: 10.1107/S0021889871006265
- J. T. Koberstein, B. Morra and R. S. Stein "The determination of diffuse-boundary thicknesses of polymers by small-angle X-ray scattering" J. Appl. Cryst. 1980, 13, 34. doi: 10.1107/S0021889880011478
- Wikipedia: Porod law
Porod fractal law
For high-q, gives specific surface area:
- References:
Structured Diffuse Scattering
Even the highest-quality single-crystal materials exhibit some level of disorder, giving rise to diffuse scattering. Single-Crystal Diffuse Scattering (SCDS) can be analyzed to understand local (short-range) ordering. Experimentally, it appears as weak and diffuse 'lines' that interconnect between the bright diffraction spots. While the diffraction spots describe the idealized unit-cell, this diffuse scattering encodes the deviation from the average structure. The diffuse scattering can be quantitatively modeled.
- T. R. Welberry and D. J. Goossens The interpretation and analysis of diffuse scattering using Monte Carlo simulation methods Acta Crystallographica Section A: Foundations and Advances 2008, 64 (1), 23-32 doi: 10.1107/S0108767307041918
- E. J. Chan, T. R. Welberry, D. J. Goossens, A. P. Heerdegen, A. G. Beasley and P. J. Chupas Single-crystal diffuse scattering studies on polymorphs of molecular crystals. I. The room-temperature polymorphs of the drug benzocaine Acta Crystallographica Section B Structural Science '2009, B65 (3), 382-392 doi: 10.1107/S0108768109015857
- T. R. Welberry and D. J. Goossens Diffuse scattering and partial disorder in complex structures IUCrJ 2014 1 (6), 550-562 doi: 10.1107/S205225251402065X
- E. J. Chan On the use of molecular dynamics simulation to calculate X-ray thermal diffuse scattering from molecular crystals J. Appl. Cryst. 2015, 48. doi: 10.1107/S1600576715013242
- A.G.A. Nisbet, G. Beutier, F. Fabrizi, B. Mosera and S. P. Collins Diffuse multiple scattering Acta Cryst. A 2015, 20-25. doi: 10.1107/S2053273314026515
More broadly, highly anisotropic and structured diffuse scattering indicates that the material exhibits some non-trivial combination of order and disorder in realspace. For instance, the disorder/defects may be ordered in some way (correlated disorder). Whereas diffuse scattering is traditionally seen as encoding structuring on a very local scale, structured diffuse scattering relates to order on intermediate size-scales.
- Ray Withers A modulation wave approach to the order hidden in disorder IUCrJ 2015, 2 (1), 74-84 doi: 10.1107/S2052252514022556
- David A. Keen & Andrew L. Goodwin The crystallography of correlated disorder Nature 2015, 521, 303-309. doi:10.1038/nature14453
Liquid Surface Diffuse Scattering
Grazing-incidence scattering can be performed on liquid interfaces. GISAXS scattering peaks may be seen if there is some kind of ordering at the interface (e.g. nanoparticles); but a pure liquid interface will, generically, not exhibit structural scattering. However, the disorder of the interface will generate diffuse scattering. In particular, the interface will fluctuate due to thermally-induced waves: i.e. capillary waves.
- Oleg Shpyrko Overview of X-ray Reflectivity and Diffuse Scattering from Liquid Surfaces
- C. Fradin, A. Braslau, D. Luzet, D. Smilgies, M. Alba, N. Boudet, K. Mecke & J. Daillant Reduction in the surface energy of liquid interfaces at short length scales Nature 2000, 403, 871-874. doi: 10.1038/35002533
Nanoscale Diffuse Scattering
- A. Fernández Herrero, M. Pflüger, J. Probst, F. Scholze and V. Soltwisch Characteristic diffuse scattering from distinct line roughnesses J. Appl. Cryst. 2017, 50, 1766-1772. doi: 10.1107/S1600576717014455
See Also
- Diffuse Scattering from ORNL
- Diffuse scattering in crystals from ETH Zurich
- P. Ehrhart The configuration of atomic defects as determined from scattering studies Journal of Nuclear Materials 1978, 6970, 200-214. doi: 10.1016/0022-3115(78)90244-1
- T. R. Welberry and D. J. Goossens Diffuse scattering and partial disorder in complex structures IUCrJ 2014 1 (6), 550-562 doi: 10.1107/S205225251402065X