Difference between revisions of "Lattice:Simple cubic"
KevinYager (talk | contribs) (Created page with "'''SC''' or '''simple cubic''' is the simples and most generic cubic lattice. ==Canonical SC== ===Symmetry=== * Crystal Family: Cubic * [http://en.wikipedia...") |
KevinYager (talk | contribs) |
||
| Line 1: | Line 1: | ||
| − | '''SC''' or '''simple cubic''' is the | + | '''SC''' or '''simple cubic''' is the simplest and most generic [[Lattice:Cubic|cubic]] [[lattice]]. |
==Canonical SC== | ==Canonical SC== | ||
| − | ===Symmetry=== | + | ===[[Symmetry]]=== |
* Crystal Family: Cubic | * Crystal Family: Cubic | ||
* [http://en.wikipedia.org/wiki/Lattice_system Crystal System]: [[Lattice:Cubic|Cubic]] | * [http://en.wikipedia.org/wiki/Lattice_system Crystal System]: [[Lattice:Cubic|Cubic]] | ||
Latest revision as of 17:48, 13 November 2016
SC or simple cubic is the simplest and most generic cubic lattice.
Contents
Canonical SC
Symmetry
- Crystal Family: Cubic
- Crystal System: Cubic
- Bravais Lattice: P (pcc)
- Crystal class: Hexoctahedral
- Point Group: m3m
- Space Group: Pm3m
- Particles per unit cell:
- Volume of unit cell:
- Dimensionality:
- Projected d-dimensional volume:
- Solid angle:
- Nearest-neighbor distance:
- Assuming spherical particles of radius R:
- Particle volume fraction:
- Maximum volume fraction: when
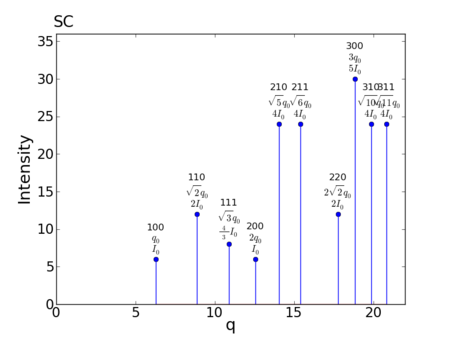
Reciprocal-Space Peaks
peak q value h,k,l m f intensity 1: 6.283185307180 1,0,0 6 1 6 2: 8.885765876317 1,1,0 12 1 12 3: 10.882796185405 1,1,1 8 1 8 4: 12.566370614359 2,0,0 6 1 6 5: 14.049629462081 2,1,0 24 1 24 6: 15.390597961942 2,1,1 24 1 24 7: 17.771531752633 2,2,0 12 1 12 8: 18.849555921539 3,0,0 30 1 30 9: 19.869176531592 3,1,0 24 1 24 10: 20.838968152189 3,1,1 24 1 24
Alternating Simple Cubic
A simple cubic lattice where the particle identity (in every direction) alternates between two possibilities. Thus this lattice has 2 distinct particles, in a 1:1 ratio. The unit cell may be thought of as having 8 particles (4 of each type).
Examples
Atomics
- Sodium chloride (NaCl) (a = 5.6402 Å)