Peak shape

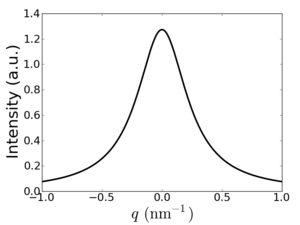
The peak width observed in x-ray scattering can be related to the grain size of the ordered structure giving rise to the scattering peak. More generally, the peak shape also encodes information about the sample order. Thus, peak shape analysis can be used to extract higher-order information.

Note also that instrumental resolution contributes to peak width, and also to peak shape. Scattering peaks are thus sometimes fit using functions that include two contributes (e.g. a Gaussian, representing material grain size, plus a Lorentzian, representing instrumental resolution).

Generalized Peak Shape

A generalized peak shape can be computed using:

${\begin{alignedat}{2}L_{hkl}(q)&={\frac {2}{\pi \delta }}\prod _{n=0}^{\infty }{\left(1+{\frac {\gamma _{\nu }^{2}}{(n+\nu /2)^{2}}}{\frac {4q_{s}^{2}}{\pi ^{2}\delta ^{2}}}\right)^{-1}}\\&={\frac {2}{\pi \delta }}\left|{\frac {\Gamma \left[\nu /2+i\gamma _{\nu }(4q_{s}^{2}/\pi ^{2}\delta ^{2})^{2}\right]}{\Gamma \left[\nu /2\right]}}\right|^{2}\end{alignedat}}$

Where $q_{s}=(q-q_{hkl})$, $\delta$ describes the peak width, and $\nu$ describes the peak shape. The parameter $\gamma _{\nu }$ is a ratio of gamma functions:

$\gamma _{\nu }={\sqrt {\pi }}{\frac {\Gamma \left[(\nu +1)/2\right]}{\Gamma \left[\nu +/2\right]}}$

The limiting cases for peak shape are:

$L_{hkl}(q_{s})=\left\{{\begin{array}{c l l}{\frac {\delta /2\pi }{q_{s}^{2}+(\delta /2)^{2}}}&\mathrm {for} \,\,\nu \to 0&\mathrm {(Lorentzian)} \\{\frac {2}{\pi \delta }}\exp \left[-{\frac {4q_{s}^{2}}{\pi \delta ^{2}}}\right]&\mathrm {for} \,\,\nu \to \infty &\mathrm {(Gaussian)} \\\end{array}}\right.$

Thus the parameter $\nu$ allows one to vary continuously between a Lorentzian peak shape and a Gaussian peak shape. Note that for Lorentzian, $\delta$ describes the full-width at half-maximum (FWHM):

$\delta _{\mathrm {lorentz} }=\mathrm {fwhm_{\mathrm {lorentz} }}$

The Gaussian form can be written a few different ways:

${\begin{alignedat}{2}L_{hkl,\mathrm {gauss} }(q_{s})&={\frac {2}{\pi \delta }}\exp \left[-{\frac {4q_{s}^{2}}{\pi \delta ^{2}}}\right]\\&={\frac {1}{{\sqrt {2\pi }}\sigma }}\exp \left[-{\frac {q_{s}^{2}}{2\sigma ^{2}}}\right]\\&={\sqrt {\frac {\ln {2}}{\pi }}}{\frac {1}{\mathrm {fwhm} }}\exp \left[-{\frac {4\ln {2}q_{s}^{2}}{\mathrm {fwhm} ^{2}}}\right]\\\end{alignedat}}$

where the width is described by:

$\delta _{\mathrm {gauss} }={\sqrt {\frac {8}{\pi }}}\sigma _{\mathrm {gauss} }={\frac {\mathrm {fwhm} _{\mathrm {gauss} }}{\sqrt {\pi \ln {2}}}}$

$\sigma _{\mathrm {gauss} }={\sqrt {\frac {\pi }{8}}}\delta _{\mathrm {gauss} }={\frac {\mathrm {fwhm} _{\mathrm {gauss} }}{2{\sqrt {2\ln {2}}}}}$

$\mathrm {fwhm} _{\mathrm {gauss} }=2{\sqrt {2\ln {2}}}\sigma _{\mathrm {gauss} }={\sqrt {\pi \ln {2}}}\delta _{\mathrm {gauss} }$

And note that $2{\sqrt {2\ln {2}}}\approx$ 2.35482004503...

Source

• Scattering Curves of Ordered Mesoscopic Materials S. Förster, A. Timmann, M. Konrad, C. Schellbach, A. Meyer, S.S. Funari, P. Mulvaney, R. Knott, J. Phys. Chem. B, 2005, 109 (4), pp 1347–1360 DOI: 10.1021/jp0467494

Literature Examples

TBD

See Also

• Scherrer grain size analysis: Converting the peak width into a measure of the structural coherence length (grain size)
• Estimating Crystallite Size Using XRD, Scott A. Speakman, MIT.