Difference between revisions of "Wave packet"
KevinYager (talk | contribs) |
KevinYager (talk | contribs) (→Position-momentum tradeoff) |
||
| Line 10: | Line 10: | ||
:<math> \tilde{\psi}(k) = \frac{1}{\sqrt{2 \pi}} \int {\psi}(x) e^{-i k x } \mathrm{d}x </math> | :<math> \tilde{\psi}(k) = \frac{1}{\sqrt{2 \pi}} \int {\psi}(x) e^{-i k x } \mathrm{d}x </math> | ||
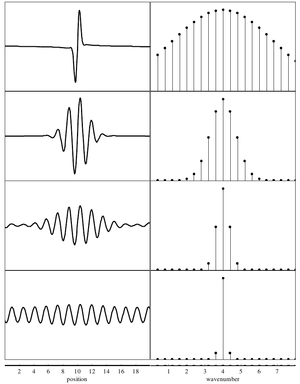
Note that these two descriptions are [[Fourier transform]]s of one another. Thus, there is an inherent tradeoff between the 'spread' of a wave-packet in position-space vs. momentum-space. | Note that these two descriptions are [[Fourier transform]]s of one another. Thus, there is an inherent tradeoff between the 'spread' of a wave-packet in position-space vs. momentum-space. | ||
| − | + | [[Image:Space tradeoff.png|center|thumb|Tradeoff between spread of a wave-packet in position-space (left) and momenum-space (right).]] | |
==Wave-particle duality== | ==Wave-particle duality== | ||
Revision as of 08:34, 13 October 2014
A wave packet is a localized wavelike perturbation, which appears frequently in quantum descriptions of particles. For instance, incident x-rays during scattering experiments can be thought of as wave-packets.
Position-momentum tradeoff
The wave-packet can be described as a wavefunction in either position-space or momentum-space:
Note that these two descriptions are Fourier transforms of one another. Thus, there is an inherent tradeoff between the 'spread' of a wave-packet in position-space vs. momentum-space.
Wave-particle duality
In the context of quantum mechanics, particles can be thought of as wave-packets. That is, quantum particles are neither ideal point-like particles, nor ideal plane-waves. They are instead intermediate objects, which are certainly wave-like (e.g. can undergo interference), but also somewhat localized. The classical concepts of 'particle' (perfectly localized; mathematically a delta-function), and 'wave' (oscillation with a single well-defined wavelength, spread infinitely over all space) can be viewed as limited cases of the general wave-packet. These limiting cases are only conceptual: in reality neither can exist.