Quantum Mechanics

Quantum mechanics is a theory that describes the interactions of all particles and systems. It underlies all physical phenomena, including scattering.

Wavefunction

A quantum system is completely specified by its Wave Function:

Integral Notation	Dirac Notation
$\psi (x)$	$\langle x|\psi \rangle$

The wavefunction is typically normalized:

Integral Notation	Dirac Notation
$\int |\psi (x)|^{2}\mathrm {d} x=1$	$\langle \psi |\psi \rangle =1$

The distribution of the particle described by $\psi (x)$ is given by:

Integral Notation	Dirac Notation
$\Pr(x)\mathrm {d} x=|\psi (x)|^{2}\mathrm {d} x$	$|\langle x|\psi \rangle |^{2}$

In the Copenhagen Interpretation, $\Pr(x)$ is the probability of finding the particle at location $x$. In Universal Wave Function interpretations (e.g. MWI), $\Pr(x)$ can be thought of as the spatial distribution of the particle. The wavefunction contains all the information one can know about a system. It can thus be thought of as 'being' the particle/system in question. However, the wavefunction can be described in an infinite number of different ways. That is, there is not a unique basis for describing the wavefunction. So, for instance, one can describe the wavefunction using position-space or momentum-space:

$\psi (x)\longleftrightarrow {\tilde {\psi }}(k)$

These representations can be inter-related (c.f. Fourier transform):

$\psi (x)={\frac {1}{\sqrt {2\pi }}}\int {\tilde {\psi }}(k)e^{ikx}\mathrm {d} k$

${\tilde {\psi }}(k)={\frac {1}{\sqrt {2\pi }}}\int {\psi }(x)e^{-ikx}\mathrm {d} x$

Example of a 1D wavefunction (plotted versus 'x'). The upper panel shows the amplitude-squared, while the lower panel shows the corresponding wavefunction components: real (black line) and imaginary (blue line).

State

Note that the wavefunction describes the state of the system; there are various choices of basis one can use as an expansion.

$\psi =\sum _{n}c_{n}\psi _{n}$

A basis should be orthonormal:

Integral Notation	Dirac Notation
$\int |\psi _{n}(x)|^{2}\mathrm {d} x=1$	$\langle \psi _{n}|\psi _{n}\rangle =1$	normalized
$\int \psi _{m}(x)^{*}\psi _{n}(x)\mathrm {d} x=0$	$\langle \psi _{m}|\psi _{n}\rangle =0$	orthogonal

An operator defines a particular convenient basis: one can always expand $\psi$ using the basis defined by an operator, in which case the $\psi _{n}$ above are the eigenvectors (or eigenstates) of that basis. This can also be viewed as a vector in the Hilbert space. The Dirac notation (bra-ket notation) is useful in this regard. A particular state is a (column) vector:

$|\psi \rangle ={\begin{bmatrix}c_{1}\\c_{2}\\\vdots \\c_{n}\end{bmatrix}}$

Which is a 'ket'. We define a 'bra' (the 'final state') as a (row) vector:

$\langle \psi |={\begin{bmatrix}c_{1}^{*}&c_{2}^{*}&\dots &c_{n}^{*}\end{bmatrix}}$

And note that the 'bra' is the conjuagte transpose of the 'ket':

$\langle \psi |^{\dagger }=|\psi \rangle$

Wave packet

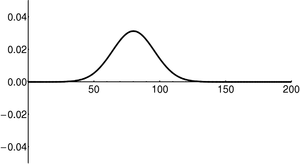
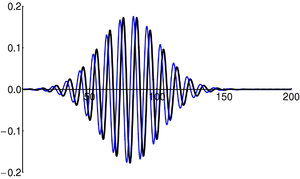
A wave packet is a localized wavelike perturbation. Particles in quantum mechanics can be thought of as wave-packets.

A 1D wave packet (with dispersion), propagating over time.

Note that "wave-particle duality" can be misleading. One can imagine a quantum particle as "both a wave and a particle"; however, it might be better to instead imagine it as a "wave packet". The 'particle' and 'wave' descriptions are really idealized limiting cases, which never appear in reality:

• A classical 'particle' is a point-like object. In QM would have a corresponding infinite spread in its momentum. Such an idealized (infinitely small) entity cannot truly exist.
• A classical 'wave' is a plane wave: an oscillation with a perfectly well-defined wavelength, extending infinitely in both directions. In QM, we indeed note that having a precisely-defined wavelength (momentum) implies infinite spatial spread (i.e. the wave fills the entire universe). Such a construct is not physically-realizable.

Heisenberg Indeterminacy Relations

(Also known as Heisenberg Uncertainty Principle.)

$\Delta _{x}\Delta _{p}\geq {\frac {\hbar }{2}}$

$\Delta _{E}\Delta _{t}\geq {\frac {\hbar }{2}}$

Superposition

If $\psi _{1}(x)$ and $\psi _{2}(x)$ are both allowed states for a given system, then the following state is also allowed:

$\psi (x)=\alpha \psi _{1}(x)+\beta \psi _{2}(x)$

This leads to a notable consequence:

${\begin{alignedat}{2}\Pr(x)&=|\alpha \psi _{1}(x)+\beta \psi _{2}(x)|^{2}\\&=(\alpha \psi _{1}+\beta \psi _{2})(\alpha \psi _{1}+\beta \psi _{2})^{*}\\&=|\alpha |^{2}|\psi _{1}|^{2}+|\beta |^{2}\psi _{2}^{2}+\alpha \beta ^{*}\psi _{1}\psi _{2}^{*}+\alpha ^{*}\beta \psi _{1}^{*}\psi _{2}\\&=\mathrm {Pr} _{1}(x)+\mathrm {Pr} _{2}(x)+\mathrm {interference} \\\end{alignedat}}$

Notice that the final terms represent 'interference' between the two constituent states. This interference has no classical analogue; it is a quantum effect. Thus a superposition is not merely a 'joining' of the two states (e.g. "the particle can be in state 1 or state 2"), but a truly coherent interference between the two states. The superposition may be more generally written as:

Integral Notation	Dirac Notation
$\int |\psi (x)|^{2}\mathrm {d} x=1$	$\langle \psi |\psi \rangle =1$

The distribution of the particle described by $\psi (x)$ is given by:

Integral Notation	Dirac Notation
$\psi (x)=\sum _{n}c_{n}\psi _{n}$	$|\psi \rangle =c_{1}|1\rangle +c_{2}|2\rangle +c_{3}|3\rangle +\cdots$

Operators

Observables in QM appears as operators (${\hat {O}}$).

Examples: TBD.

Measurement

The transition of the wavefunction $\psi$ into state $\phi$ can be thought of as:

$\int \phi ^{*}\psi \mathrm {d} x$

$\langle \phi |\psi \rangle =a_{1}^{*}c_{1}+a_{2}^{*}c_{2}+a_{3}^{*}c_{3}+\cdots$

When acting on a wavefunction with operator ${\hat {O}}$ the probability that the wavefunction ends up in state $\phi _{n}$ is given by:

${\begin{alignedat}{2}\Pr(O_{n})&=\left|\int \phi _{n}^{*}\psi \mathrm {d} x\right|^{2}\\&=\left|\int \phi _{n}^{*}\sum _{m}c_{m}\phi _{m}\mathrm {d} x\right|^{2}\\&=\left|c_{n}\int \phi _{n}^{*}\phi _{n}\mathrm {d} x\right|^{2}\\&=|c_{n}|^{2}\end{alignedat}}$

${\begin{alignedat}{2}\Pr(O_{n})&=|\langle n|\psi \rangle |^{2}\\&=|c_{n}|^{2}\end{alignedat}}$

The solutions take the form of an eigenvalue problem:

${\hat {O}}\phi _{n}=o_{n}\phi _{n}$

The allowed solutions of the equation, for operator ${\hat {O}}$, involve an eigenstate $\phi _{n}$ with associated eigenvalue $o_{n}$. A measurement on a quantum system can be thought of as driving the wavefunction into an eigenstate defined by the operator; the value of the associated observable is then fixed to be the corresponding eigenvalue. (As noted above, the probability of ending up in a particular eigenstate is regulated by the coefficient of that eigenstate in the original wavefunction decomposition.)

Expectation value

A given operator, e.g. ${\hat {A}}$, implies an expectation value (for state $\psi$) of:

$\langle A\rangle _{\psi }=\int \psi ^{*}{\hat {A}}\psi \mathrm {d} x$

$\langle A\rangle _{\psi }=\langle \psi |{\hat {A}}|\psi \rangle$

If the system is in an eigenstate of the operator:

$\psi =\sum _{n}c_{n}\psi _{n}=\psi _{n}$

We know that:

${\hat {A}}\psi _{n}=a_{n}\psi _{n}$

And so:

${\begin{alignedat}{2}\langle A\rangle &=\int \psi _{n}^{*}{\hat {A}}\psi _{n}\mathrm {d} x\\&=\int \psi ^{*}a_{n}\psi \mathrm {d} x\\&=a_{n}\int \psi ^{*}\psi \mathrm {d} x\\&=a_{n}\\\end{alignedat}}$

In other words, the expectation value of an eigenstate is simply the eigenvalue.

Schrödinger Equation

Time-independent equation

This simplified version of the Schrödinger equation can be used to solve for allowed stationary states. The general form is akin to the eigenvalue problems noted above: the energy operator (${\hat {H}}$) acts on the system state ($\Psi$) to yield an energy eigenvalue ($E$):

$E\Psi ={\hat {H}}\Psi$

For a single non-relativistic particle, the Hamiltonian is known and the Schrödinger equation takes the form:

$E\Psi (\mathbf {r} )=\left[{\frac {-\hbar ^{2}}{2m}}\nabla ^{2}+V(\mathbf {r} )\right]\Psi (\mathbf {r} )$

Time-dependent equation

More generally, the time-evolution of the wavefunction should be considered. The full version of the Schrödinger equation thus includes time dependence:

$i\hbar {\frac {\partial }{\partial t}}\Psi ={\hat {H}}\Psi$

Again for a single non-relativistic particle, we can write more specifically that:

$i\hbar {\frac {\partial }{\partial t}}\Psi (\mathbf {r} ,t)=\left[{\frac {-\hbar ^{2}}{2\mu }}\nabla ^{2}+V(\mathbf {r} ,t)\right]\Psi (\mathbf {r} ,t)$

Entanglement

When systems (or Hilbert spaces) A and B interact, they become entangled. Before the interaction, the two systems are simply a composite system:

$|\psi \rangle _{A}\otimes |\phi \rangle _{B}$

At this level, states are separable. However, the composite system more generally should be written as:

$|\psi \rangle _{AB}=\sum _{i,j}c_{ij}|i\rangle _{A}\otimes |j\rangle _{B}$

If all the coefficients can be written as $\scriptstyle c_{ij}=c_{i}^{A}c_{j}^{B},$, then there is no coupling between the two states. We call the system separable, since it can be decomposed into the two sub-systems:

$|\psi \rangle _{A}=\sum _{i}c_{i}^{A}|i\rangle _{A}$

$|\phi \rangle _{B}=\sum _{j}c_{j}^{B}|j\rangle _{B}$

However, if $\scriptstyle c_{ij}\neq c_{i}^{A}c_{j}^{B}$, then the states are non-separable, or entangled.

The entanglement of an observer (or simply measurement apparatus) can also be considered. For observer $\scriptstyle |\psi \rangle$ performing measurement $\scriptstyle U$ on system $\scriptstyle \sum a_{n}|S_{n}\rangle$:

$U(|\psi \rangle \otimes |S_{n}\rangle )=|\psi _{n}\rangle \otimes |S_{n}\rangle$

This leads to the evolution:

$|\psi \rangle \otimes \sum a_{n}|S_{n}\rangle \to \sum a_{n}|\psi _{n}\rangle \otimes |S_{n}\rangle$

Where the right-hand-side describes an entanglement between the observer and the system being studied.

Density Matrices

The outer product of a ket with a bra defines a 2D matrix; i.e. a linear operator.

$|\phi \rangle \langle \psi |={\begin{bmatrix}\phi _{1}\\\phi _{2}\\\vdots \\\phi _{N}\end{bmatrix}}{\begin{bmatrix}\psi _{1}^{*}&\psi _{2}^{*}&\cdots &\psi _{N}^{*}\end{bmatrix}}={\begin{bmatrix}\phi _{1}\psi _{1}^{*}&\phi _{1}\psi _{2}^{*}&\cdots &\phi _{1}\psi _{N}^{*}\\\phi _{2}\psi _{1}^{*}&\phi _{2}\psi _{2}^{*}&\cdots &\phi _{2}\psi _{N}^{*}\\\vdots &\vdots &\ddots &\vdots \\\phi _{N}\psi _{1}^{*}&\phi _{N}\psi _{2}^{*}&\cdots &\phi _{N}\psi _{N}^{*}\end{bmatrix}}$

Density matrices can be a useful way to visualize the interactions between states of a system. For instance, a pure state is given by a density matrix that has only a single non-zero term, along the diagonal:

$|\psi \rangle \langle \psi |={\begin{bmatrix}1&0\\0&0\end{bmatrix}}$

By comparison, a mixed state is one where more than one diagonal term is non-zero, but off-diagonal terms are zero:

$|\psi \rangle \langle \psi |={\begin{bmatrix}0.5&0\\0&0.5\end{bmatrix}}$

The diagonal terms of the density matrix will be real numbers (and can be interpreted as probabilities). The above matrix describes a classical mixture of different states. In the Copenhagen interpretation, this would be described as a situation where the system will randomly collapse into one of the possible states. Under MWI, it would instead be said that the various states are now non-interacting, and thus will evolve independently.

The most general case is where the off-diagonal terms are non-zero. These terms represent the interference aspects. Thus this describes an entangled state:

$|\psi \rangle \langle \psi |={\begin{bmatrix}0.36&-0.48i\\+0.48i&0.64\end{bmatrix}}$

The off-diagonal terms indicate the strength and nature of the interference between the states. During decoherence, these off-diagonal terms are driven towards zero.

Decoherence

TBD

See Also

• Wikipedia: Quantum Mechanics