Difference between revisions of "Reflectivity"
KevinYager (talk | contribs) |
KevinYager (talk | contribs) (→Mathematical form) |
||
| Line 28: | Line 28: | ||
R_S = \frac{ r_{01} + r_{12} e^{ 2i \tilde{k}^1_z h } }{ 1 + r_{01} r_{12} e^{ 2i \tilde{k}^1_z h } } | R_S = \frac{ r_{01} + r_{12} e^{ 2i \tilde{k}^1_z h } }{ 1 + r_{01} r_{12} e^{ 2i \tilde{k}^1_z h } } | ||
</math> | </math> | ||
| − | where <math>\tilde{k}^j_z=-\sqrt | + | where |
| + | :<math>\tilde{k}^j_z = - \sqrt{ n^2_j k^2_0 - |k_{\parallel}|^2 }</math> | ||
| + | is the perpendicular component of the wave-vector (in medium ''j''). The reflectivity coefficients are: | ||
:<math> | :<math> | ||
f_{ij} = \frac{ \tilde{k}^i_z - \tilde{k}^j_z }{ \tilde{k}^i_z + \tilde{k}^j_z } | f_{ij} = \frac{ \tilde{k}^i_z - \tilde{k}^j_z }{ \tilde{k}^i_z + \tilde{k}^j_z } | ||
</math> | </math> | ||
| − | Where <math>\scriptstyle r_{01}</math> and <math>\scriptstyle r_{12}</math> for the [[vacuum]]-layer and layer-substrate interfaces, respectively. This is called the 'one-box model'. | + | Where <math>\scriptstyle r_{01}</math> and <math>\scriptstyle r_{12}</math> for the [[Material:Vacuum|vacuum]]-layer and layer-substrate interfaces, respectively. This is called the 'one-box model'. |
===Parratt formalism=== | ===Parratt formalism=== | ||
TBD | TBD | ||
| − | |||
==See Also== | ==See Also== | ||
Revision as of 11:46, 28 January 2015
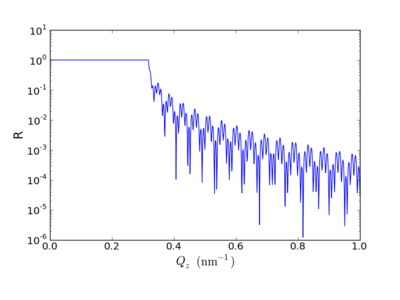
Reflectivity refers to the measurement of the intensity of reflection off of a flat interface. The term both describes the physical phenomenon, as well as the experimental technique.
X-ray Reflectivity (XRR or XR) and neutron reflectivity (NR) are techniques which measure the intensity of reflected radiation as a function of angle (where, by definition for specular reflectivity, the incident and exit angles are equal; ). A plot of reflectivity (R) versus angle yields the reflectivity curve. For XR and NR, the data is typically plotted as a function of the momentum transfer parallel to the film normal:
Off-Specular Reflectivity
TBD
Mathematical form
In its simplest form, the Fresnel reflectivity can be given by:
Where:
And n is the complex refractive index of the substrate. The idealized uncorrelated roughness can be characterized by a mean standard deviation of the height :
For a substrate with a single continuous layer of thickness h (e.g. a uniform thin film), the reflectivity becomes:
where
is the perpendicular component of the wave-vector (in medium j). The reflectivity coefficients are:
Where and for the vacuum-layer and layer-substrate interfaces, respectively. This is called the 'one-box model'.
Parratt formalism
TBD
See Also
- Fresnel plot
- Oscillations below the critical angle
- DWBA: A formalism for modeling GISAXS data, including reflection effects.