Difference between revisions of "Wave packet"
KevinYager (talk | contribs) (→Wave-particle duality) |
KevinYager (talk | contribs) (→Propagation and Interaction) |
||
| Line 19: | Line 19: | ||
{| | {| | ||
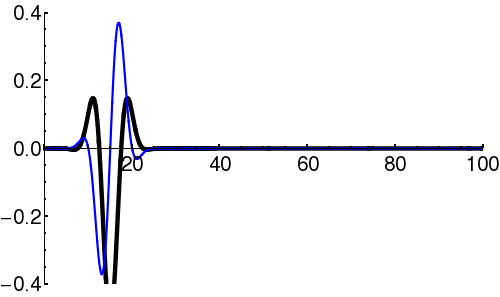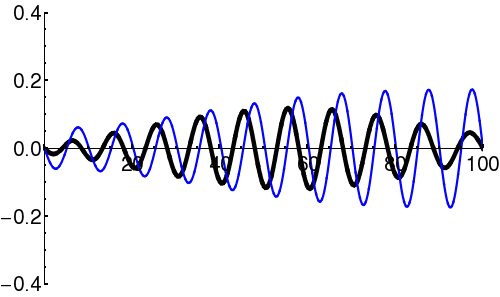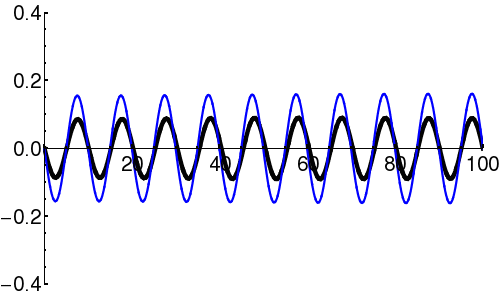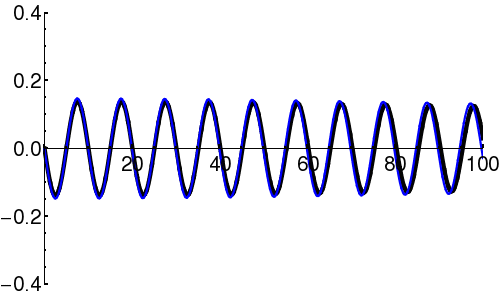
| − | | [[Image:Wavepacket-Box- | + | | [[Image:Wavepacket-Box-wavefunc3.gif|500px|thumb|Components of the wavefunction (<math>\scriptstyle \psi(x) </math>) describing a wave-packet bouncing inside a box-like potential. The black line is the real part, and the blue line is the imaginary part.]] |
|- | |- | ||
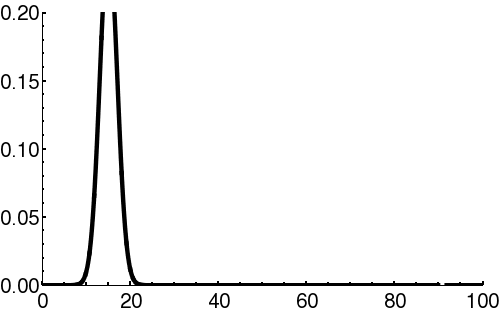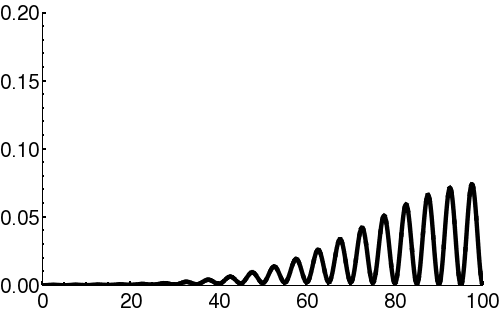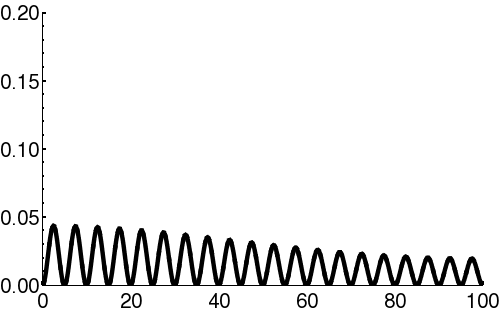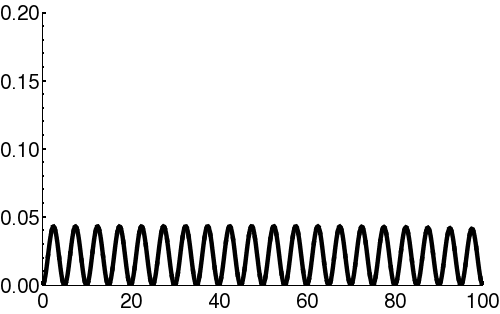
| [[Image:Wavepacket-Box-sqr2.gif|500px|thumb|Square of the wavefunction (<math>\scriptstyle | \psi(x) |^2 </math>).]] | | [[Image:Wavepacket-Box-sqr2.gif|500px|thumb|Square of the wavefunction (<math>\scriptstyle | \psi(x) |^2 </math>).]] | ||
Revision as of 08:32, 3 November 2014
A wave packet is a localized wavelike perturbation, which appears frequently in quantum descriptions of particles. For instance, incident x-rays during scattering experiments can be thought of as wave-packets.
Contents
Position-momentum tradeoff
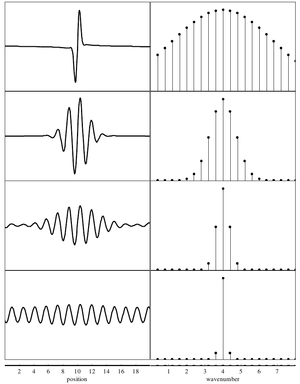
The wave-packet can be described as a wavefunction in either position-space or momentum-space:
Note that these two descriptions are Fourier transforms of one another. Thus, there is an inherent tradeoff between the 'spread' of a wave-packet in position-space vs. momentum-space.
Wave-particle duality
In the context of quantum mechanics, particles can be thought of as wave-packets. That is, quantum particles are neither ideal point-like particles, nor ideal plane-waves. They are instead intermediate objects, which are certainly wave-like (e.g. can undergo interference), but also somewhat localized. The classical concepts of 'particle' (perfectly localized; mathematically a delta-function), and 'wave' (oscillation with a single well-defined wavelength, spread infinitely over all space) can be viewed as limited cases of the general wave-packet. These limiting cases are only conceptual: in reality neither can exist.
Propagation and Interaction
Wave-packets can propagate, frequently with dispersion (which induces spatial spread of the envelope). Wave-packets can interact with barriers (defined by, e.g., some potential function), and interfere with themselves.