Reflectivity

Reflectivity refers to the measurement of the intensity of reflection off of a flat interface. The term both describes the physical phenomenon, as well as the experimental technique.

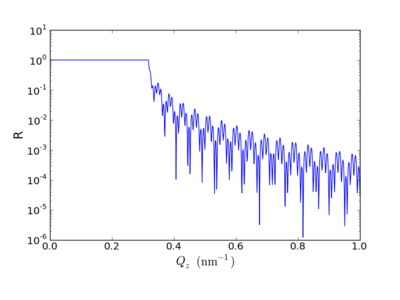
X-ray Reflectivity (XRR or XR) and neutron reflectivity (NR) are techniques which measure the intensity of reflected radiation as a function of angle (where, by definition for specular reflectivity, the incident and exit angles are equal; $\scriptstyle \theta _{i}=\theta _{f}$). A plot of reflectivity (R) versus angle yields the reflectivity curve. For XR and NR, the data is typically plotted as a function of the momentum transfer parallel to the film normal:

$q_{z}={\frac {4\pi }{\lambda }}\sin \theta _{i}$

Reflectivity calculation for a thin film.

Off-Specular Reflectivity

TBD

Mathematical form

Ideal interface

In its simplest form, the Fresnel reflectivity can be given by:

$r_{F}={\frac {k_{z}-{\tilde {k}}_{z}}{k_{z}+{\tilde {k}}_{z}}}$

Where $\scriptstyle k_{z}$ is the vertical (z) component of the momentum of the incident wave, and $\scriptstyle {\tilde {k}}_{z}$ is the vertical component of the transmitted wave:

${\tilde {k}}_{z}=-{\sqrt {n^{2}k_{0}^{2}-|k_{\parallel }|^{2}}}$

and n is the complex refractive index of the substrate. The reflected intensity (which is what is measured experimentally) is $R_{F}=|r_{f}|^{2}$. Close to the critical angle ($\scriptstyle \theta _{i}\approx \theta _{c}$), the beam is strongly refracted and:

${\tilde {k}}_{z}=k{\sqrt {\theta _{i}-\theta _{c}}}$

Thus, when the incident angle is less than the critical angle ($\scriptstyle \theta _{i}<\theta _{c}$), the transmitted wave-vector is purely imaginary: \tilde{k}_z = i k \sqrt{ \theta_c - \theta_i } This corresponds to the wave being totally externally reflected (evanescent probing only). Above the critical angle, the reflected intensity falls off rapidly: $R_{F}\sim \theta _{i}^{-4}$ (c.f. Fresnel plot).

Rough interface

A flat interface with a small amount of roughness can be modelled using idealized uncorrelated roughness, which is characterized by a mean standard deviation of the height $\scriptstyle \sigma ={\sqrt {\left\langle h^{2}\right\rangle }}$:

$r_{S}=r_{F}e^{-2\sigma ^{2}k_{z}{\tilde {k}}_{z}}$

For a substrate with a single continuous layer of thickness h (e.g. a uniform thin film), the reflectivity becomes:

$r_{S}={\frac {r_{01}+r_{12}e^{2i{\tilde {k}}_{z}^{1}h}}{1+r_{01}r_{12}e^{2i{\tilde {k}}_{z}^{1}h}}}$

where

${\tilde {k}}_{z}^{j}=-{\sqrt {n_{j}^{2}k_{0}^{2}-|k_{\parallel }|^{2}}}$

is the perpendicular component of the wave-vector (in medium j). The reflectivity coefficients are:

$f_{ij}={\frac {{\tilde {k}}_{z}^{i}-{\tilde {k}}_{z}^{j}}{{\tilde {k}}_{z}^{i}+{\tilde {k}}_{z}^{j}}}$

Where $\scriptstyle r_{01}$ and $\scriptstyle r_{12}$ for the vacuum-layer and layer-substrate interfaces, respectively. This is called the 'one-box model'.

Multiple interfaces: Parratt formalism

The reflectivity from a stack of different layers can be computed using the so-called Parratt recursion formalism, which accounts for the effect of reflection from each internal interface, including their coherent interference to yield the final reflection intensity. This formalism can, in fact, be used to simulate or fit an arbitrary electron-density distribution (in the film-normal direction). Any continuous variation of density can be simulated by discretizing the system into a finite number of layers of finite thickness.

The Parratt formalism is recursive. The reflected and transmitted amplitude of layer j are given by $\scriptstyle R_{j}$ and $\scriptstyle T_{j}$, such that:

$X_{j}={\frac {R_{j}}{T_{j}}}=e^{-2ik_{z,j}z_{j}}{\frac {r_{j,j+1}+X_{j+1}e^{2ik_{z,j}z_{j}}}{1+r_{j,j+1}X_{j+1}e^{2ik_{z,j}z_{j}}}}$

where

$r_{j,j+1}={\frac {k_{z,j}-k){z,j+1}}{k_{z,j}+k){z,j+1}}}$

is the Fresnel coefficient for interface $\scriptstyle j$. The resultant system of equations can be solved by setting $T_{1}=1$ (i.e. the incident wave is normalized to unity) and $R_{N+1}=0$ (i.e. there is no reflection from the bottom of the substrate).

The interference between various reflections generically leads to oscillations in the reflectivity curve (so-called Kiessig fringes). A one-box model (uniform thin film on a substrate) will give rise to a set of fringes with a single periodicity, which is related to the film thickness h via $\Delta q_{z}=2\pi /h$. Multiple layers of different thickness will give fringes of different periodicities, superimposed in the reflectivity curve. This can also give rise to beat-patterns in the curve. A multi-layer stack with a repeated thickness (e.g. alternating layers of two materials, always with the same thickness) will interfere constructively to give a strong peak at a q_z corresponding to the layering distance. This is called a Bragg peak, and can also be thought of as the Structure Factor of lamellar ordering.

There are many available software packages which implement the Parratt formalism. These packages can be used to fit experimental data, and thereby obtain a possible (thought not unambiguous) curve for the realspace average density (SLD) profile in the film-normal direction.

• L. G. Parratt Surface Studies of Solids by Total Reflection of X-Rays Phys. Rev. 1954, 95, 359. doi: 10.1103/PhysRev.95.359

Master-Formula

For sufficiently large incident angle ($\scriptstyle \theta _{i}\gg \theta _{c}$), a kinematical appoximation can be valid. In such a case, the electron-density profile $\scriptstyle \rho _{e}(z)$ can be described using the so-called Master-Formula:

$R(q_{z})=R_{F}\left|{\frac {1}{\rho _{e}(z\to \infty )}}\int {\frac {\mathrm {d} \rho _{e}}{\mathrm {d} z}}e^{iq_{z}z}\mathrm {d} z\right|^{2}$

This formalism breaks down near the critical angle, but can be useful for predicting the reflectivity at higher angles, since the closed-form equation is simple to evaluate. It should be noted that this is essentially a Fourier transform of the density variation. In fact, this form highlights that the reflectivity comes from the variation of the material's refractive index (only points with non-zero $\mathrm {d} \rho _{e}/\mathrm {d} z$ contribute to the integral). This makes plain that the reflectivity comes from locations where the refractive-index changes abruptly (at interfaces), or gradually.

• P. Fenter, F. Schreiber, V. Bulovic, and S. R. Forrest Thermally induced failure mechanism of organic light emitting device structures probed by X-ray specular reflectivity Chem. Phys. Lett. 1997, 277, 521.
• W. Hamley and J. S. Pedersen Analysis of neutron and X-ray reflectivity J. Appl. Cryst. 1994, 27, 29.

See Also

• Fresnel plot
• Oscillations below the critical angle
• DWBA: A formalism for modeling GISAXS data, including reflection effects.