Difference between revisions of "Refraction distortion"
KevinYager (talk | contribs) |
KevinYager (talk | contribs) (→Mathematics) |
||
| Line 5: | Line 5: | ||
==Mathematics== | ==Mathematics== | ||
The GISAXS refraction distortion shifts the data along <math>\scriptstyle q_z</math>, leaving <math>\scriptstyle q_x</math> unaffected. The amount of the shift is given by: | The GISAXS refraction distortion shifts the data along <math>\scriptstyle q_z</math>, leaving <math>\scriptstyle q_x</math> unaffected. The amount of the shift is given by: | ||
| + | :<math> | ||
| + | \begin{alignat}{2} | ||
| + | \Delta q_z & = q_z - q_z^{\prime} \\ | ||
| + | q'_z & = k_0\left(\sqrt{\sin\alpha_i^2 - \sin\alpha_{ct}^2} + \sqrt{\sin\alpha_f^2 - \sin\alpha_{ct}^2}\right) \\ | ||
| + | & = k_0\left(\sqrt{\sin\alpha_i^2 - \sin\alpha_{ct}^2} + \sqrt{\left(\frac{q_z}{k_0} - \sin\alpha_i\right)^2 - \sin\alpha_{ct}^2}\right) | ||
| − | [[Image:Lu GTSAXS-Figure5.png|center|thumb| | + | \end{alignat} |
| + | </math> | ||
| + | Where <math>\scriptstyle \alpha_i</math> is the incident angle, and <math>\scriptstyle \alpha_{ct}</math> is the [[critical angle]] of the film. | ||
| + | |||
| + | [[Image:Lu GTSAXS-Figure5.png|center|thumb|450px|Figure from Lu. et al. ([http://dx.doi.org/10.1107/S0021889812047887 doi: 10.1107/S0021889812047887 ''J. of Appl. Cryst.'' '''2013''', 46, 165]) showing the amount of distortion along ''q<sub>z</sub>'', for different conditions.]] | ||
==Refraction Correction== | ==Refraction Correction== | ||
Revision as of 13:24, 4 November 2015
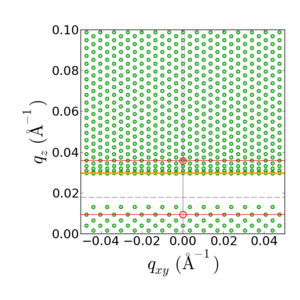
Illustration of GISAXS refraction distortion. The reciprocal-space scattering is a hexagonal array of peaks. However, these peaks are both shifted, and compressed/stretched along qz, due to refraction. This effect is especially pronounced near the Yoneda (orange line).
In GISAXS, GIWAXS, and other grazing-incidence techniques, the refractive index difference between the film and the ambient causes the incident and scattered x-ray beams to be refracted. This extent of refraction depends on the incident and exit angles. Thus, the data that appears on an area detector in a grazing-incidence experiment is non-linearly distorted. This makes data interpretation more problematic.
Mathematics
The GISAXS refraction distortion shifts the data along , leaving unaffected. The amount of the shift is given by:
Where is the incident angle, and is the critical angle of the film.
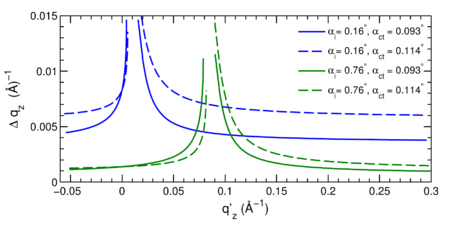
Figure from Lu. et al. (doi: 10.1107/S0021889812047887 J. of Appl. Cryst. 2013, 46, 165) showing the amount of distortion along qz, for different conditions.
Refraction Correction
When computing theoretical scattering patterns, one must account for the refraction correction.
- Byeongdu Lee, Insun Park, Jinhwan Yoon, Soojin Park, Jehan Kim, Kwang-Woo Kim, Taihyun Chang, and Moonhor Ree Structural Analysis of Block Copolymer Thin Films with Grazing Incidence Small-Angle X-ray Scattering Macromolecules 2005, 38 (10), 4311-4323. doi: 10.1021/ma047562d
- P. Busch, M. Rauscher, D.-M. Smilgies, D. Posselt and C. M. Papadakis Grazing-incidence small-angle X-ray scattering from thin polymer films with lamellar structures - the scattering cross section in the distorted-wave Born approximation J. Appl. Cryst. 2006, 39, 433-442. doi: 10.1107/S0021889806012337
- Rémi Lazzari, Frédéric Leroy, and Gilles Renaud Grazing-incidence small-angle x-ray scattering from dense packing of islands on surfaces: Development of distorted wave Born approximation and correlation between particle sizes and spacing Phys. Rev. B 2007, 76, 125411. doi: 10.1103/PhysRevB.76.125411
- D. W. Breiby, O. Bunk, J. W. Andreasen, H. T. Lemke and M. M. Nielsen Simulating X-ray diffraction of textured films J. Appl. Cryst. 2008, 41, 262-271. doi: 10.1107/S0021889808001064
- Lu, X.; Yager, K.G.; Johnston, D.; Black, C.T.; Ocko, B.M. Grazing-incidence transmission X-ray scattering: surface scattering in the Born approximation Journal of Applied Crystallography 2013, 46, 165–172. doi: 10.1107/S0021889812047887
See Also
- DWBA
- Simulating X-ray diffraction of textured films D. W. Breiby, O. Bunk, J. W. Andreasen, H. T. Lemke and M. M. Nielsen J. Appl. Cryst. 2008, 41, 262-271. doi: 10.1107/S0021889808001064
- Indexation scheme for oriented molecular thin films studied with grazing-incidence reciprocal-space mapping D.-M. Smilgies and D. R. Blasini J. Appl. Cryst. 2007, 40, 716-718. doi: 10.1107/S0021889807023382
- Grazing-incidence small-angle X-ray scattering from thin polymer films with lamellar structures - the scattering cross section in the distorted-wave Born approximation P. Busch, M. Rauscher, D.-M. Smilgies, D. Posselt and C. M. Papadakis J. Appl. Cryst. 2006, 39, 433-442. doi: 10.1107/S0021889806012337




