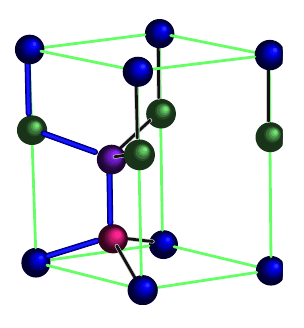
Lattice:Hexagonal diamond
The hexagonal diamond lattice is an arrangement of tetrahedrally-bonded elements, within a hexagonal unit cell. Whereas conventional diamond (a.k.a. cubic diamond) exists within a cubic unit cell, hexagonal diamond exists within a hexagonal unit cell. In both cases, elements are bonded tetrahdrally. However, in cubic diamond, the six-membered rings are all in the chair conformation, whereas in hexagonal diamond, some six-membered rings are in the boat conformation.
Contents
Canonical Hexagonal Diamond
A canonical hexagonal diamond lattice (single atom/particle type arranged as shown above) has symmetry Fd3m.
Symmetry
- Crystal Family: Hexagonal
- Crystal System: Hexagonal
- Bravais Lattice: hexagonal
- Crystal class: Hexoctahedral
- Space Group: P63/mmc
- Particles per unit cell: Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle n=4}
- Volume of unit cell: Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle V_d=a^2 c \sin(60^{\circ}) = a^2 c \frac{\sqrt{3}}{2}}
- Dimensionality: Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle d=3}
Structure
TBD
Particle Positions
There are 14 positions. In total there are 4 particles in the unit cell.
Fractional
Positions are given in terms of fractional coordinates relative to the unit-cell edge-vectors:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{a}=(a,0,0)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{b}=(b/2,\sqrt{3}b/2,0)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{c}=(0,0,c)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{bottom\,\, layer}: \, \frac{1}{12} + \frac{1}{6} + \frac{1}{12} + \frac{1}{6} = \frac{1}{2}}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,0\right),(1,0,0),(0,1,0),(1,1,0)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{mid\,\, layer}: \, \frac{1}{6} + \frac{1}{3} + \frac{1}{6} + \frac{1}{3} = 1}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,\frac{5}{8}\right),\left(1,0,\frac{5}{8}\right),\left(0,1,\frac{5}{8}\right),\left(1,1,\frac{5}{8}\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2 \, \mathrm{internal\,\, strut}: \, 1 + 1 = 2}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(\frac{1}{3},\frac{1}{3},\frac{1}{8}\right),\left(\frac{1}{3},\frac{1}{3},\frac{4}{8}\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{top\,\, layer}: \, \frac{1}{12} + \frac{1}{6} + \frac{1}{12} + \frac{1}{6} = \frac{1}{2}}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,1\right),(1,0,1),(0,1,1),(1,1,1)}
Absolute
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{bottom\,\, layer}: \, \frac{1}{12} + \frac{1}{6} + \frac{1}{12} + \frac{1}{6} = \frac{1}{2}}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,0\right),(a,0,0),\left(\frac{b}{2},\frac{\sqrt{3}b}{2},0\right),\left(a+\frac{b}{2},\frac{\sqrt{3}b}{2},0\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{mid\,\, layer}: \, \frac{1}{6} + \frac{1}{3} + \frac{1}{6} + \frac{1}{3} = 1}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,\frac{5c}{8}\right),\left(a,0,\frac{5c}{8}\right),\left(\frac{b}{2},\frac{\sqrt{3}b}{2},\frac{5c}{8}\right),\left(a+\frac{b}{2},\frac{\sqrt{3}b}{2},\frac{5c}{8}\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2 \, \mathrm{internal\,\, strut}: \, 1 + 1 = 2}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(\frac{a}{2},\frac{b}{2\sqrt{3}},\frac{c}{8}\right),\left(\frac{a}{2},\frac{b}{2\sqrt{3}},\frac{4c}{8}\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{top\,\, layer}: \, \frac{1}{12} + \frac{1}{6} + \frac{1}{12} + \frac{1}{6} = \frac{1}{2}}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,c\right),(a,0,c),\left(\frac{b}{2},\frac{\sqrt{3}b}{2},c\right),\left(a+\frac{b}{2},\frac{\sqrt{3}b}{2},c\right)}
Distances
For a particle-particle bond-length of Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle l} :
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a=\frac{2\sqrt{6}}{3}l}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=a=\frac{2\sqrt{6}}{3}l}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle c=\frac{8}{3}l}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{a}{c}=\frac{\sqrt{6}}{4}\approx 0.61237}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \frac{c}{a}=\frac{4}{\sqrt{6}}\approx 1.63299}
Absolute (in terms of particle-particle distance)
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{bottom\,\, layer}: \, \frac{1}{12} + \frac{1}{6} + \frac{1}{12} + \frac{1}{6} = \frac{1}{2}}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,0\right),\left(\frac{2\sqrt{6}}{3}l,0,0 \right),\left(\frac{\sqrt{6}}{3}l,\sqrt{2}l,0\right),\left(\sqrt{6}l,\sqrt{2}l,0\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{mid\,\, layer}: \, \frac{1}{6} + \frac{1}{3} + \frac{1}{6} + \frac{1}{3} = 1}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,\frac{5}{3}l\right),\left(\frac{2\sqrt{6}}{3}l,0,\frac{5}{3}l \right),\left(\frac{\sqrt{6}}{3}l,\sqrt{2}l,\frac{5}{3}l\right),\left(\sqrt{6}l,\sqrt{2}l,\frac{5}{3}l\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2 \, \mathrm{internal\,\, strut}: \, 1 + 1 = 2}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(\frac{\sqrt{6}}{3}l,\frac{\sqrt{2}}{3}l,\frac{1}{3}l\right),\left(\frac{\sqrt{6}}{3}l,\frac{\sqrt{2}}{3}l,\frac{4}{3}l\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{top\,\, layer}: \, \frac{1}{12} + \frac{1}{6} + \frac{1}{12} + \frac{1}{6} = \frac{1}{2}}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,\frac{8}{3}l\right),\left(\frac{2\sqrt{6}}{3}l,0,\frac{8}{3}l \right),\left(\frac{\sqrt{6}}{3}l,\sqrt{2}l,\frac{8}{3}l\right),\left(\sqrt{6}l,\sqrt{2}l,\frac{8}{3}l\right)}
Examples
Atomics
- Lonsdaleite form of carbon (C), also known as hexagonal diamond, 2H diamond, or 'sp3 diamond' (a = 2.51 Å, c = 4.12 Å)
Alternating Hexagonal Diamond
This is the Wurtzite crystal structure, a hexagonal unit cell with alternating species.
Examples
Atomics
- Wurtzite (Zn,Fe)S (a = b = 3.82 Å, c = 6.26 Å)
Along Connections
This lattice can be thought of as the hexagonal-dimaond analog of the cubic-diamond cristobalite. Here, a four-bonded species occupies all the sites of the canonical hexagonal diamond lattice, and a two-bonded species sits along each of the connections between these tetrahedral sites.
Particle Positions
Particle Type A (bond tetrahedrally)
These are the same positions as the canonical hexagonal diamond.
Particle Type B (two-fold bonded)
There are 11 positions. In total there are 8 particles of this type in the unit cell.
Fractional
Positions are given in terms of fractional coordinates relative to the unit-cell edge-vectors:
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{a}=(a,0,0)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{b}=(b/2,\sqrt{3}b/2,0)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathbf{c}=(0,0,c)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 3 \, \mathrm{lower\,\,tripod}: \, 1 + 1 + 1 = 3}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(\frac{1}{6},\frac{1}{6},\frac{1}{16}\right),\left(\frac{4}{6},\frac{1}{6},\frac{1}{16}\right),\left(\frac{1}{6},\frac{4}{6},\frac{1}{16}\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1 \, \mathrm{mid\,\,strut}: \, 1 = 1}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(\frac{1}{3},\frac{1}{3},\frac{5}{16}\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 3 \, \mathrm{midlevel\,\,tripod}: \, 1 + 1 + 1 = 3}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(\frac{1}{6},\frac{1}{6},\frac{9}{16}\right),\left(\frac{4}{6},\frac{1}{6},\frac{9}{16}\right),\left(\frac{1}{6},\frac{4}{6},\frac{9}{16}\right)}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 4 \, \mathrm{upper\,\,connections}: \, \frac{1}{6} + \frac{1}{3} + \frac{1}{6} + \frac{1}{3} = 1}
- Failed to parse (MathML with SVG or PNG fallback (recommended for modern browsers and accessibility tools): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \left(0,0,\frac{13}{16}\right),\left(1,0,\frac{13}{16}\right),\left(0,1,\frac{13}{16}\right),\left(1,1,\frac{13}{16}\right)}